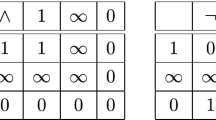
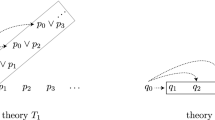
Abstract
This paper discusses the general problem of translation functions between logics, given in axiomatic form, and in particular, the problem of determining when two such logics are “synonymous” or “translationally equivalent.” We discuss a proposed formal definition of translational equivalence, show why it is reasonable, and also discuss its relation to earlier definitions in the literature. We also give a simple criterion for showing that two modal logics are not translationally equivalent, and apply this to well-known examples. Some philosophical morals are drawn concerning the possibility of having two logical systems that are “empirically distinct” but are both translationally equivalent to a common logic.
Similar content being viewed by others
REFERENCES
Birkhoff, G. (1967): Lattice Theory, Amer. Math. Soc. Colloq. Publ. 25.
Chellas, B. F. (1980): Modal Logic: An Introduction, Cambridge University Press, Cambridge.
Czelakowski, J. (1980): Equivalential logics I, II, Studia Logica 40, 227–236, 335–372.
Da Silva, J., D'Ottaviano, M., and Sette, A. (1999): Translations between logics, in X. Casedo and C. Montenegro (eds), Models, Algebras and Proofs, Marcel Dekker, New York, pp. 435–448.
De Bouvère, K. (1965): Synonymous theories, in J. Addison, L. Henkin and A. Tarski (eds), Symposium on the Theory of Models, North-Holland, pp. 402–406.
Epstein, R. (1990): The Semantic Foundations of Logic. Vol. 1: Propositional Logics, Kluwer Academic Publishers, Dordrecht.
Hughes, G. and Cresswell, M. (1996): A New Introduction to Modal Logic, Routledge.
Jónsson, B. and Tarski, A. (1951): Boolean algebras with operators. Part I, Amer. J. Math. 73, 891–939.
Jónsson, B. and Tarski, A. (1952): Boolean algebras with operators. Part II, Amer. J. Math. 74, 127–162.
Kanger, S. (1968): Equivalent theories, Theoria 34, 1–6.
Kirk, R. (1982): On three alleged rivals to homophonic translation, Philos. Stud. 42, 409–418.
Kleene, S. (1952): Introduction to Metamathematics, Van Nostrand, Princeton.
Krajíček, J. (1996): Bounded Arithmetic, Propositional Logic and Complexity Theory, Cambridge University Press.
Kuhn, S. T. (1977): Many-sorted Modal Logics, Uppsala University Filosofiska Studier Number 29.
Lenzen, W. (1979): Epistemologische Betrachtungen zu [S4,S5], Erkenntnis 14, 33–56.
Massey, G. (1978): Indeterminacy, inscrutability, and ontological relativity, in Studies in Ontology, Amer. Philosophical Quarterly Monograph Ser. 9, Basil Blackwell.
McKinsey, J. and Tarski, A. (1948): Some theorems about the sentential calculi of Lewis and Heyting, J. Symbolic Logic 13, 1–15.
Montague, R. M. (1957): Contributions to the axiomatic foundations of set theory, PhD thesis, University of California, Berkeley.
Pelletier, F. J. (1984a): The not-so-strange modal logic of indeterminacy, Logique et Anal. 108, 415–422.
Pelletier, F. J. (1984b): Six problems in “translational equivalence”, Logique et Anal. 108, 423–434.
Pollard, S. (1998): Homeomorphism and the equivalence of logical systems, Notre Dame J. Formal Logic 39, 422–435.
Prawitz, D. and Malmnäs (1968): A survey of some connections between classical, intuitionistic and minimal logic, in H. Schmidt, K. Schütte and H.-J. Thiele (eds), Contributions to Mathematical Logic, North-Holland, pp. 215–229.
Prior, A. (1967): Past, Present and Future, Oxford University Press.
Prucnal, T. and Wroński, A. (1974): An algebraic characterization of the notion of structural completeness, Bull. Sec. Logic Polish Acad. Sci. 3, 45–52.
Quine, W. (1960): Word and Object, MIT Press.
Segerberg, K. (1982): Classical Propositional Operators, Clarendon Press, Oxford.
Sobociński, B. (1964): Modal system S4.4, Notre Dame J. Formal Logic 5, 305–312.
Thomason, S. (1974): Reduction of tense logic to modal logic I, J. Symbolic Logic 39, 549–551.
Thomason, S. (1975): Reduction of tense logic to modal logic II, Theoria 3, 154–169.
Troelstra, A. S. and van Dalen, D. (1988): Constructivism in Mathematics: An Introduction, North-Holland, Amsterdam.
White, N. (ed.) (1986): Theory of Matroids, Cambridge University Press.
Wójcicki, R. (1988): Theory of Logical Calculi, Kluwer Academic Publishers, Dordrecht.
Zeman, J. J. (1971): A study of some systems in the neighbourhood of S4.4, Notre Dame J. Formal Logic 12, 341–357.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Pelletier, F.J., Urquhart, A. Synonymous Logics. Journal of Philosophical Logic 32, 259–285 (2003). https://doi.org/10.1023/A:1024248828122
Issue Date:
DOI: https://doi.org/10.1023/A:1024248828122