Hostname: page-component-8448b6f56d-sxzjt Total loading time: 0 Render date: 2024-04-19T10:35:01.003Z Has data issue: false hasContentIssue false
  • English
  • Français

More about uniform upper bounds on ideals of turing degrees1

Published online by Cambridge University Press:  12 March 2014

Harold T. Hodes
Harold T. Hodes*
Affiliation:
Department of Philosophy, Cornell University, Ithaca, New York 14853
Get access Rights & Permissions [Opens in a new window]

Abstract

Let I be a countable jump ideal in 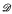= 〈The Turing degrees, ≤〉. The central theorem of this paper is:

a is a uniform upper bound on I iff a computes the join of an I-exact pair whose double jump a(1) computes.

We may replace “the join of an I-exact pair” in the above theorem by “a weak uniform upper bound on I”.

We also answer two minimality questions: the class of uniform upper bounds on I never has a minimal member; if ⋃I = Lα[A] ⋂ ωω for α admissible or a limit of admissibles, the same holds for nice uniform upper bounds.

The central technique used in proving these theorems consists in this: by trial and error construct a generic sequence approximating the desired object; simultaneously settle definitely on finite pieces of that object; make sure that the guessing settles down to the object determined by the limit of these finite pieces.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 48 , Issue 2 , June 1983 , pp. 441 - 457
Copyright
Copyright © Association for Symbolic Logic 1983

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

1

I wish to thank David Posner for an illuminating discussion which led to all these theorems.

References

BIBLIOGRAPHY

[1]Hensel, G. and Putnam, H., On the notational independence of various hierarchies of degrees of unsohability, this Journal, vol. 30 (1965), pp. 64–86.Google Scholar
[2]Hodes, H., Uniform upper bounds on ideals of Turing degrees, this Journal, vol. 43 (1978), pp. 601–612.Google Scholar
[3]Jockusch, C. and Simpson, S., A degree-theoretic characterization of the ramified analytical hierarchy, Annals of Mathematical Logic, vol. 10 (1976), pp. 1–32.CrossRefGoogle Scholar