Abstract
We defend hylomorphism against Maegan Fairchild’s purported proof of its inconsistency. We provide a deduction of a contradiction from SH+, which is the combination of “simple hylomorphism” and an innocuous premise. We show that the deduction, reminiscent of Russell’s Paradox, is proof-theoretically valid in classical higher-order logic and invokes an impredicatively defined property. We provide a proof that SH+ is nevertheless consistent in a free higher-order logic. It is shown that the unrestricted comprehension principle of property abstraction on which the purported proof of inconsistency relies is analogous to naïve unrestricted set-theoretic comprehension. We conclude that logic imposes a restriction on property comprehension, a restriction that is satisfied by the ramified theory of types. By extension, our observations constitute defenses of theories that are structurally similar to SH+, such as the theory of singular propositions, against similar purported disproofs.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
Notes
Fine (1982) is explicit that a qua-object exists only when the base has the form (p. 100). Fairchild does not specify what, if anything, ⌜α/Π⌝ is supposed to mean when α designates x, Π designates F, and x lacks F. Either way, the qua-function is undefined when x lacks F.
The word ‘qua’, which putatively designates a particular function from individuals and properties to qua-objects, is as we use it, an “indirect” (ungerade) operator in that the occurrence of ‘Michelle’s husband’ in ‘Barack qua Michelle’s husband’ designates not its default (or “customary”) designatum, Barack, but its indirect designatum, the property of being Michelle’s husband. The slash designates the very same function as ‘qua’, but it is not an indirect operator. (In Fairchild’s alternate use ‘qua’, like ‘/’, is also not indirect. Where we say ‘Barack qua Michelle’s husband’ and ‘m qua statue-shaped’, she would say, deviating from Standard English-cum-Latin usage, ‘Barack qua being Michelle’s husband’ and ‘m qua being statue-shaped’).
The symbol ‘λ’ is a variable-binding operator that forms a compound functional expression from an open expression. Strictly speaking, the lambda-abstract ⌜λα[ξα]⌝ designates the function that assigns to any value of α the designatum of ξα under the assignment of that value to α. In the special case of an open formula ϕα, ⌜λα[ϕα]⌝ is a compound monadic predicate. For the purposes of this paper, we follow common practice in taking a monadic predicate to designate a property (namely, the one that corresponds to the relevant propositional function that is, strictly speaking, designated). In English, this corresponds to the formation of the compound monadic predicate ⌜is a thing such that ϕit⌝ from the open formula ϕit in which the pronoun ‘it’ functions as a free variable, for example, the formation of ‘is a thing such that it is snub-nosed and it is wise’ (in short, ‘is snub-nosed and wise’) from ‘it is snub-nosed and it is wise’. Such lambda-abstracts are governed by inference rules of lambda-conversion. In particular, lambda-expansion (property abstraction) permits the inference from the formula ϕβ to ⌜λα[ϕα]β⌝, where ϕβ is the result of uniformly substituting free occurrences of the singular term β for the free occurrences of the singular-term variable α in ϕα. In English, this corresponds to the inference from ‘Socrates is snub-nosed and Socrates is wise’ to ‘Socrates is snub-nosed and wise’. Fairchild appears to treat the properties over which the monadic-predicate variables range as intensions, that is, as functions from possible worlds to extensions (p. 35n10). We do not here object to treating predicates as designating intensions. Given this understanding of the entities over which the monadic-predicate variables range, a lambda-abstract ⌜λα[ϕα]⌝ is definable by means of the second-order definite description ⌜
Π□∀α(Πα ↔ ϕα)⌝ where Π is a monadic-predicate variable. Compare Church (1974a, p. 30). Alternatively, the definite-description operator ‘
’ is definable in terms of lambda-abstraction together with an appropriate higher-level function. (So that, for example, ⌜
αϕα)⌝ is definable by means of ⌜Ιλα[ϕα]⌝ where ‘Ι’ is the function that assigns to any property the only individual that has that property if such exists, and is undefined otherwise.) Lambda-abstraction underlies all variable binding and is therefore more basic than definite-description formation.First-order free-logical UI (∀-Elim) licenses the inference from ⌜∀αϕα⌝ and the supplementary premise ⌜∃γ(γ = β)⌝ to ϕβ, where the variable γ does not occur free in the singular term β and ϕβ is the result of uniformly substituting free occurrences of β for the free occurrences of the variable α in ϕα. First-order free-logical EG (∃-Intro) licenses the inference from ϕβ and the same supplementary premise ⌜∃γ(γ = β)⌝ to ⌜∃αϕα⌝. First-order free-logic also involves corresponding modifications of ∀-Intro and ∃-Elim. As we use the term here, free second-order logic analogously modifies the classical logic of the quantifiers to take account of monadic predicates that do not designate any element of the universe over which the monadic-predicate variables range.
Reflection on SH reveals that the example by which Fairchild explains the guiding idea of hylomorphism is oversimplified. Let m be the marble that constitutes Michelangelo’s David and let F be its specific shape. Even if m qua statue-shaped has F as David does, it, unlike David, presumably could have had a very different shape. So it is a mistake to identify David with m qua statue-shaped. Compare Fine (1982), which identifies Goliath with Goliath-matter qua Goliath-shaped and not with Goliath-matter qua statue-shaped. Even the specific shape (or suitable variations thereon) is probably not enough. Even if m/F is so-shaped, it could have come to have F entirely by natural forces instead of by design. Arguably, David is essentially a sculpted statue not sculpted primarily by anyone other than Michelangelo. If so, then, not only is it incorrect to identify David with m qua statue-shaped, it is even incorrect to identify David with m/F. Compare Fairchild (2017, p. 34n5).
It is by no means clear that hylomorphists are prepared to regard the form of a statue as a modal intension. On a more standard understanding of a property, and correspondingly of lambda-abstraction, there are infinitely many witnesses for an instance of MPC, all of which determine the same intension. For example, being a qua-object that both embodies a property it lacks and is such that the number one is odd is another witness for MPCS distinct from S.
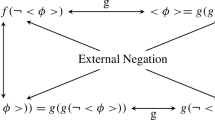
The term ‘stone-caster’ derives from John 8:7: “Let s/he who is without sin cast the first stone.” We shall later question the legitimacy of the definition of the term ‘stone-caster’ as a term for a special kind of qua-object. This will have the effect of also questioning much of the discussion to follow that incorporates ‘stone-caster’ as potentially meaningless. “My propositions serve as elucidations in the following way: anyone who understands me eventually recognizes them as nonsensical, when he has used them—as steps—to climb up beyond them. (He must, so to speak, throw away the ladder after he has climbed up it.) He must transcend these propositions, and then he will see the world aright” (Wittgenstein [1922] 2018, 6.54).
Fairchild gives an intricate argument for the claim. We offer (without hereby endorsing) instead a simple alternative: if there is any non-qua-object m, then there is a property (not being a qua-object) that m qua non-qua-object (that is, m/not being a qua-object) embodies and also lacks. Where our alternative relies on there being an instantiated property of not being a qua-object (in conjunction with Existence), her argument relies on the plausible assumptions that there are at least two individuals, a and b, and that there are at least three properties, one had only by a, one had only by b, and one had only by a and b (in conjunction with Existence and Uniqueness). Both arguments are compelling. Fairchild’s argument will be more appealing than our alternative to an SH proponent who, like Sosa (1999), thinks that it is mere dogma to suppose that there are non-qua-objects (p. 141).
Our rendering of Fairchild’s argument corrects reasoning and other errors in both her informal prose (pp. 35–36) and her “more rigorous” presentation (pp. 35–36n10, 14, and 15).
Compare Church (1974a), pp. 29–30. The following is a (schematic) proof of PC in classical second-order logic with lambda-abstraction.
1.
∀x(ϕx ↔ ϕx)
logic (ϕx is any formula without free ‘F’.)
2.
∀x[ λy[ϕy](x) ↔ ϕx ]
1, lambda-expansion, logic
3.
∃F∀x(Fx ↔ ϕx)
2, 2nd-order existential generalization on ⌜λy[ϕy]⌝
See note 3. A similar schematic argument may be given for MPC in classical second-order logic with lambda-abstraction and modality. This argument assumes as an additional premise that the lambda-abstract is a rigid designator.
That is, the ∈–Pair Interpretation may be regarded as being obtained in two stages. First, the singular-term variables are taken as ranging over the pure sets, while the monadic-predicate variables are taken as ranging over classes of pure sets and ‘λ’ is interpreted as an operator for class abstraction. The slash is interpreted as a symbol for the ordered-pair operation. Already at this stage the slash stands for a partial function, undefined when its second argument is a proper class, since proper classes are not elements of ordered pairs. The interpretation obtained at the first stage satisfies both PC and Uniqueness, but Existence fails for any instance in which ‘F’ is assigned a proper class. At the second stage the slash is restricted to ∈-pairs and (more important) the proper classes of the first stage are excised, so that the monadic-predicate variables now range over “small classes” of pure sets and any lambda-abstract that designates a proper class at the first stage is stripped of its designatum and therewith of its semantic extension.
The notation ‘{G}’ is a first-order singular term for a set. It may be taken as defined by ‘
x∀F(F ∈2x ↔ F =2 G)’, where ‘∈2’ is a dyadic predicate for a relation between a property of individuals and an individual. As we use it, ‘
’ validates the schema ⌜Π(…
αϕα …) ↔ ∃β[∀α(ϕα ↔ α = β) & Π(… β …)⌝ where α and β are distinct individual variables and Π is a simple predicate.The analog of Uniqueness, namely
- Uniqueness*:
∀F∀G[{F} = {G} ↔ F =2 G],
is a logical consequence of Existence*. (Refer to note 12.) Fairchild is sympathetic to overcoming her challenge to SH by rejecting Uniqueness while retaining Existence (pp. 38–39). There is no analogous option for the Paradox of Property Singletons.
Salmón (unpublished) provides a fuller treatment of the Paradox of Property Singletons.
By saying that PC “generates” properties we mean merely that it posits for any open formula a corresponding property.
This notion of impredicativity is a special case of, but stricter than, the broader notion, largely based on Henri Poincaré’s vicious-circle principle (1906), to wit, that of introducing a particular element of a class by quantifying over the elements of that class. Although it is not impredicatively defined in the stricter sense, in this broader sense the putative set involved in Russell’s Paradox (the set of all and only those sets that are not elements of themselves) is said to be “impredicatively defined.” However, as F. P. Ramsey in effect pointed out (1925, p. 204), so also is the idea of fixing reference by a superlative definite description, for example, ‘the shortest spy’, ‘the first child to be born in the 22nd Century’, etc. (We thank C. Anthony Anderson for supplying this reference.) The stricter sense of ‘impredicative’, which is likely what is usually meant in the relevant literature, is uniformly adhered to throughout the present essay.
The notion of definition by abstraction involved in the stricter notion of impredicativity is to be sharply distinguished from the distinct notion in the neo-logicism literature that bears the same label.
According to this response, the lambda-abstract abbreviated by ‘S’ does not designate on the intended interpretation of SH, consequently ‘∀F’ in Existence cannot be legitimately instantiated to it. The free second-order logic employed here requires the supplementary premise ‘∃F(F = 2 λy[ϕy])’ for lambda-expansion, thus rendering the inference at line 2 in the derivation in note 10 fallacious. The missing supplementary premise is itself a property-comprehension schema.
See Church (1976), Russell (1903, Appendix B), Russell (1908), and Whitehead and Russell (1927) 1963, *12, pp. 161–167. Whitehead and Russell’s axioms of reducibility entail that every level n property for n ≥ 1 is co-extensive with a level 1 property. It is sometimes said—following a highly misleading (at best) remark of Quine (1967, p. 152)—that the axioms of reducibility restore the paradoxes of impredicativity. The claim is incorrect, however, as Russell had already noted in 1908 (last paragraph of section V). See Church (1974b, p. 356).
Where ϕx invokes quantification over properties of individuals the proof of PC in note 10 thereby invokes the basic form of impredicative definition. By contrast, impredicative PC is not a theorem of ramified type theory. In a suitable formulation of ramified type theory it is not even well-formed.
The proposal for restricting Existence that Fairchild discusses on pp. 37–38 bears a superficial similarity to the present suggestion but is radically different.
The use of the plural “problems” is misleading, since it suggests that Fairchild provides considerations against SH in addition to her Russellian challenge.
We take the force of Fairchild’s “may be” to be to leave open the possibility that SH + is “unsafe” for reasons unrelated to her argument. The fact of this concession renders the interpretation of Fairchild’s argument that we offer in §3 exceedingly plausible in spite of its poor fit with her tagline that SH is inconsistent.
Π□∀α(Πα ↔ ϕα)⌝ where Π is a monadic-predicate variable. Compare Church (References
Church, A. (1974a). Russellian simple type theory. Proceedings and Addresses of the American Philosophical Association,47, 21–33.
Church, A. (1974b). Review of Quine 1971. Journal of Symbolic Logic,39(2), 355–356.
Church, A. (1976). Comparison of Russell’s resolution of the semantical antinomies with that of Tarski. Journal of Symbolic Logic,41(4), 747–760.
Fairchild, M. (2017). A paradox of matter and form. Thought,6, 33–42.
Fine, K. (1982). Acts, events, and things. In Language and ontology, 97–105 as part of the proceedings of the sixth international wittgenstein symposium 23rd to 30th August 1981, Kirchberg/Wechsel (Austria). Vienna: Holder-Pichler-Tempsky.
Johnston, M. (2006). Hylomorphism. Journal of Philosophy,103(12), 652–698.
Koslicki, K. (2008). The structure of objects. Oxford: Oxford University Press.
Kripke, S. (1979). A puzzle about belief. In A. Margalit (Ed.), Meaning and use (pp. 239–283). Boston: D. Reidel.
Poincaré, H. (1906). Les Mathématiques et la logique. Revue de Métaphysique et de Morale,14, 294–317.
Quine, W. V. O. 1967 (1971). Introduction to Russell 1908. In van Heijenoort J (ed.) From Frege to Gödel: A source book in mathematical logic, 1879–1931 (pp. 150–152). Harvard University Press.
Ramsey, F. P. (1925). The foundations of mathematics. In F. P. Ramsey (Ed.) Philosophical PAPERS (pp. 164–224). Cambridge University Press, Cambridge, 1990.
Rea, M. C. (2011). Hylomorphism reconditioned. Philosophical Perspectives,25(1), 341–358.
Russell, B. (1903). Principles of mathematics. Cambridge: Cambridge University Press.
Russell, B. (1908). Mathematical logic as based on the theory of types. American Journal of Mathematics,30, 222–262.
Salmón, N. (forthcoming). “Russell’s law.” To appear in a festschrift for David Kaplan edited by Joseph Almog and Jessica Pepp.
Salmón, N. (unpublished). A paradox about sets of properties.
Sosa, E. (1999). Existential relativity. Midwest Studies in Philosophy,23(1), 132–143.
Whitehead, A. N. & Russell, B. (1927) 1963. Principia mathematica. 2nd ed. Cambridge University Press, Cambridge.
Wittgenstein, L. (1922) 2018. Tractatus logico-philosophicus. Side-by-Side-by-Side Edition, Version 0.53 (February 5, 2018), containing original German, alongside both the Ogden/Ramsey and the Pears/McGinness translations. Accessed at https://people.umass.edu/klement/tlp/. 8 July 2018.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
We are indebted to C. Anthony Anderson, Daniel Z. Korman, and Saul Kripke for discussion.
Rights and permissions
About this article
Cite this article
Robertson Ishii, T., Salmón, N. Some highs and lows of hylomorphism: on a paradox about property abstraction. Philos Stud 177, 1549–1563 (2020). https://doi.org/10.1007/s11098-019-01274-4
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11098-019-01274-4