Abstract
There are decision problems in which rational deliberation fails to result in choosing a pure act. This phenomenon is known as decision instability and has been discussed in the literature on causal decision theory. In this paper we investigate another type of instability, called structural instability in dynamical systems theory. Structural instability indicates that certain qualitative features of the process of rational deliberation are under-determined in a decision situation. We illustrate some of the issues arising from structural instability with a recent argument against causal decision theory proposed by Caspar Hare and Brian Hedden. We show that their argument is undermined by considerations arising from decision instability and structural instability.






Similar content being viewed by others
Notes
See, e.g., Katok and Hasselblatt (1997, 68ff.).
For more information we refer the reader to Joyce’s insightful discussion in Joyce (2012). In a recent paper, Armendt (2019) develops a different view of rational deliberation that puts less emphasis on arriving at a state of full information. We think that Armendt provides an interesting account, but we’ll work with the more idealized picture here for the sake of simplicity.
The same goes for other decision problems in which acts are in some sense unstable.
See Hare and Hedden (2016, ft. 17).
This procedure only works under rather restrictive conditions. Both players must have knowledge of the structure of the game. For instance, the row player must know her own payoffs, but also the strategies and payoffs of her opponent; for otherwise she could not conclude that the column player will not choose Red. In order to arrive at this conclusion, she also needs to know that her opponent eliminates weakly dominated strategies. Iterating the procedure also requires players to have higher-order knowledge of the structure of the game and the elimination procedures. That is, they need to know that the other player knows their strategies, payoffs, and the fact that they eliminate weakly dominated strategies.
There is another, slightly different, problem. Assuming the agent believes with probability one that the predictor has predicted C, she has no preference among the acts. But it is also assumed that the predictor can predict with certainty that the agent will choose Crate C. What facts about the agent would such a predictor have to know? Since it cannot be anything about the preferences of the agent, we are left in the dark.
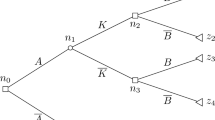
Let us illustrate order effects in terms of the following game:
$$\begin{aligned} \begin{array}{l|cc} & \hbox {Left} & \hbox {Right} \\ \hline \hbox {Up} & \$1, \$1 & \$0, \$0 \\ \hbox {Middle} & \$1, \$1 & \$2, \$1 \\ \hbox {Down} & \$0, \$0 & \$2, \$1 \\ \end{array} \end{aligned}$$If the row player applies weak dominance on Down, then the column player will eliminate Right by weak dominance, securing a payoff of \(\$ 1\) for the row player. If instead the row player applies weak dominance on Up, then the column player will eliminate Left by weak dominance, securing a payoff of \(\$ 2\) for the row player. These maneuvers fit the schematic form of weak dominance Hare and Hedden give, and so we’re faced with the curiosity that different orders of deduction yield different outcomes.
The models will utilize simplified payoffs for greater visibility in the diagrams. This induces no qualitative change in the dynamics. The new payoffs we’ll use: \(\left({\begin{matrix} 10 & 11 & 0 \\ 0 & 0 & 1 \\ 0 & 0 & 0 \\ \end{matrix}}\right)\). All calculations are carried out to a minimum of 500 digits of precision. In particular, each stage of the deliberation is calculated symbolically and then rounded to 500 digits.
This dynamics is better known as the replicator dynamics in game theory. Skyrms (1990) shows that the Darwin map is a simple instance of a Bayesian learning dynamics.
See Skyrms (1990, p. 37). If \(U(A_i)\) is the causal expected utility of \(A_i\) and if \(p_i\) denote the status quo choice probabilities, then \(U(SQ) = \sum _i p_i U(A_i)\).
Let \(P_A\) denote the proposition that the predictor predicted A and \(C_A\) the proposition that the agent chooses A, and let \({\mathbb P}\) be the agent’s probability. Then \({\mathbb P}(P_A) = {\mathbb P}(C_A)\) is equivalent to \({\mathbb P}(P_A | C_A) = {\mathbb P}(C_A | P_A)\) (whenever the relevant unconditional probabilities are non-zero). Thus the ideal predictor assumption amounts to saying that the propositions \(C_A\) and \(P_A\) depend on one another in a strictly symmetric way. This need not be the case, but it is also not implausible.
Cf. Figure 8 of Hare and Hedden (2016, p. 616) for their simpler assessment of the dynamics. Formally, the simplex is the set \(\{\langle p,q,r\rangle \in {\mathbb {R}}^3: p,q,r \ge 0, p+q+r=1\}\).
See Skyrms (1990, pp. 30–31).
See Camerer et al. (2004).
The status quo, SQ, is a probability distribution over acts. According to the ideal predictor assumption the probability distribution carries over to states. The best response to SQ is an act that maximizes expected utility with respect to that distribution. It’s possible that a best response may not be unique. For the purpose of calculating the dynamics with multiple best responses, we let BR(SQ) be the uniform mixed act of those strategies. Nothing of substance depends on that assumption.
See Hofbauer and Sigmund (1998, p. 94) for details.
Structural stability can, of course, be defined more precisely. We limit ourselves to a restricted treatment for the Three Crates problem here and leave a more thorough analysis to future work. For mathematical details on structural stability see, e.g., Guggenheimer and Holmes (1983).
More formally, the reason has to do with the eigenvalues of a deliberational equilibrium. If they take on specific values (for discrete-time dynamics 1, for continuous-time dynamics 0), then linear terms don’t determine the stability properties of the equilibrium. Small changes to the dynamics typically change the eigenvalues, however, leading to discontinuous changes in the stability properties of an equilibrium.
There are some details one needs to consider in a careful analysis. For instance, for the Darwin map, the process must start in the interior of state space.
This leads to the idea of the trembling hand. Selten (1975) argues that “a satisfactory interpretation of equilibrium points in extensive games seems to require that the possibility of mistakes is not completely excluded” (p. 15). A rational agent can never be sure to implement her intentions perfectly. Her hand might “tremble”, causing her to actually choose an act other than the one she intended to choose. Or there might be an external shock, like an earthquake, that interferes with her plan. In the Three Crates problem, this would mean that Hare and Hedden’s fourth assumption—that the agent will not choose a crate that she is certain she will not choose—can be easily violated by a rational decision maker. We don’t think that being certain that one will be able to implement one’s intention is a requirement of rationality. In fact, the opposite is true: believing with certainty that one will be able to implement one’s intentions is highly implausible. Perturbations of the trembling-hands sort are not only compatible with the agent’s epistemic and pragmatic rationality, they are required once we take into account the possibility of mistakes or external shocks.
This could, for instance, be achieved by perturbing the payoffs such that there is a small positive payoff for C in case the predictor predicted C.
Hare and Hedden (2016, p. 617).
Hare and Hedden (2016, p. 617).
There is an issue here besides the stability of an equilibrium. One could also challenge this claim by questioning premise 4 from Hare–Hedden’s original argument, namely that if a rational agent must pick an act that she is certain she will pick. If rational deliberation gets an agent to a belief state rather than an act selection, then why should arriving at full probability on a single act imply that an agent will choose that act? This inference involves a substantive step that is in need of justification, as illustrated by trembling hand considerations.
See Harper (1986) on mixed acts.
Hare and Hedden (2016, p. 622).
References
Armendt, B. (2019). Causal decision theory and decision instability. The Journal of Philosophy, 116(5), 263–277. https://doi.org/10.5840/jphil2019116517
Arntzenius, F. (2008). No regrets, or: Edith Piaf revamps decision theory. Erkenntnis, 68, 277–297.
Camerer, C. F., Ho, T.-H., & Chong, J.-K. (2004). A cognitive hierarchy model of games. The Quarterly Journal of Economics, 119(3), 861–898. https://doi.org/10.1162/0033553041502225
Gibbard, A., & Harper, W. (1978). Counterfactuals and two kinds of expected utility. In A. Hooker, J. J. Leach, & E. F. McClennen (Eds.), Foundations and applications of decision theory (pp. 125–162). Dordrecht: D. Reidel.
Guggenheimer, J., & Holmes, P. (1983). Nonlinear oscillations, dynamical systems, and bifurcations of vector fields. New York: Springer.
Hare, C., & Hedden, B. (2016). Self-reinforcing and self-frustrating decisions. Noûs, 50(3), 604–628.
Harper, W. D. (1986). Mixed strategies and ratifiability in causal decision theory. Erkenntnis, 24, 25–36.
Hofbauer, J., & Sigmund, K. (1998). Evolutionary games and population dynamics. Cambridge: Cambridge University Press.
Joyce, J. M. (2012). Regret and instability in causal decision theory. Synthese, 187, 123–145.
Katok, A., & Hasselblatt, B. (1995). Introduction to the modern theory of dynamical systems (Vol. 54). Cambridge University Press.
Selten, R. (1975). Reexamination of the perfectness concept for equilibrium points in extensive games. International Journal of Game Theory, 4(1), 25–55.
Skyrms, B. (1990). The dynamics of rational deliberation. Cambridge: Harvard University Press.
Acknowledgements
We would like to thank Gerard Rothfus, Hannah Rubin, Brian Hedden, Jim Joyce, Brian Skyrms, and Brad Armendt, as well as two anonymous reviewers, for helpful comments on an earlier version of this paper. We would also like to thank the participants of the 2018 meeting of the Society for Exact Philosophy for their comments on the presentation of this article.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Lauro, G., Huttegger, S.M. Structural Stability in Causal Decision Theory. Erkenn 87, 603–621 (2022). https://doi.org/10.1007/s10670-019-00210-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10670-019-00210-6